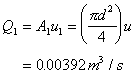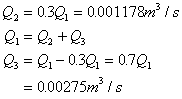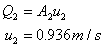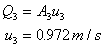1. Mass flow rate

If we want to measure the rate at which water is flowing along a pipe. A very simple way of doing this is to catch all the water coming out of the pipe in a bucket over a fixed time period. Measuring the weight of the water in the bucket and dividing this by the time taken to collect this water gives a rate of accumulation of mass. This is know as the mass flow rate.

For example an empty bucket weighs 2.0kg. After 7 seconds of collecting water the bucket weighs 8.0kg, then: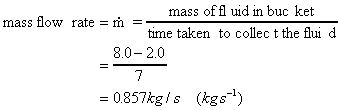

Performing a similar calculation, if we know the mass flow is 1.7kg/s, how long will it take to fill a container with 8kg of fluid?

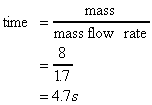
2. Volume flow rate - Discharge.

More commonly we need to know the volume flow rate - this is more commonly know as discharge. (It is also commonly, but inaccurately, simply called flow rate). The symbol normally used for discharge is Q. The discharge is the volume of fluid flowing per unit time. Multiplying this by the density of the fluid gives us the mass flow rate. Consequently, if the density of the fluid in the above example is 850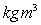then:

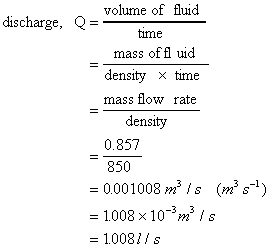
An important aside about units should be made here:

As has already been stressed, we must always use a consistent set of units when applying values to equations. It would make sense therefore to always quote the values in this consistent set. This set of units will be the SI units. Unfortunately, and this is the case above, these actual practical values are very small or very large (0.001008m³/s is very small). These numbers are difficult to imagine physically. In these cases it is useful to use derived units, and in the case above the useful derived unit is the litre.
(1 litre = 1.0 10^-3m³). So the solution becomes 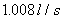. It is far easier to imagine 1 litre than 1.0 10^-3m³. Units must always be checked, and converted if necessary to a consistent set before using in an equation.

3. Discharge and mean velocity

If we know the size of a pipe, and we know the discharge, we can deduce the mean velocity

Discharge in a pipe

If the area of cross section of the pipe at point X is A, and the mean velocity here is 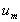. During a time t, a cylinder of fluid will pass point X with a volume A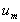t. The volume per unit time (the discharge) will thus be

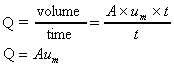
So if the cross-section area, A, is and the discharge, Q is 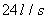, then the mean velocity, 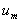, of the fluid is

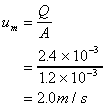
Note how carefully we have called this the mean velocity. This is because the velocity in the pipe is not constant across the cross section. Crossing the centreline of the pipe, the velocity is zero at the walls increasing to a maximum at the centre then decreasing symmetrically to the other wall. This variation across the section is known as the velocity profile or distribution. A typical one is shown in the figure below.

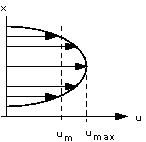
A typical velocity profile across a pipe

This idea, that mean velocity multiplied by the area gives the discharge, applies to all situations - not just pipe flow.

4. Continuity

Matter cannot be created or destroyed - (it is simply changed in to a different form of matter). This principle is know as the conservation of mass and we use it in the analysis of flowing fluids.

The principle is applied to fixed volumes, known as control volumes (or surfaces), like that in the figure below:

An arbitrarily shaped control volume.

For any control volume the principle of conservation of mass says

Mass entering per unit time = Mass leaving per unit time + Increase of mass in the control volume per unit time

For steady flow there is no increase in the mass within the control volume, so

For steady flow

Mass entering per unit time = Mass leaving per unit time

This can be applied to a streamtube such as that shown below. No fluid flows across the boundary made by the streamlines so mass only enters and leaves through the two ends of this streamtube section.

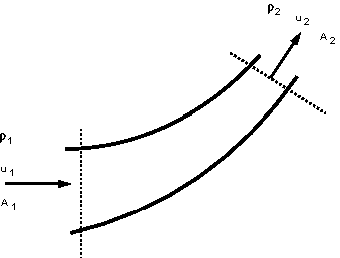
A streamtube

We can then write

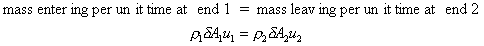
Or for steady flow,

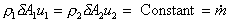
This is the equation of continuity.

The flow of fluid through a real pipe (or any other vessel) will vary due to the presence of a wall - in this case we can use the mean velocity and write

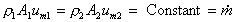
When the fluid can be considered incompressible, i.e. the density does not change, r₁ = r₂ = r so (dropping the m subscript)

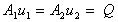
This is the form of the continuity equation most often used.

This equation is a very powerful tool in fluid mechanics and will be used repeatedly throughout the rest of this course.

Some example applications

We can apply the principle of continuity to pipes with cross sections which change along their length. Consider the diagram below of a pipe with a contraction:

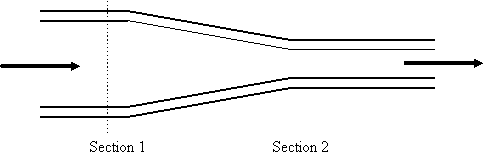
A liquid is flowing from left to right and the pipe is narrowing in the same direction. By the continuity principle, the mass flow rate must be the same at each section - the mass going into the pipe is equal to the mass going out of the pipe. So we can write:

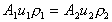
(with the sub-scripts 1 and 2 indicating the values at the two sections)

As we are considering a liquid, usually water, which is not very compressible, the density changes very little so we can say . This also says that the volume flow rate is constant or that

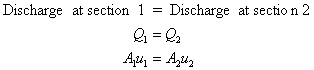
For example if the area 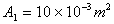and 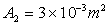and the upstream mean velocity, 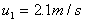, then the downstream mean velocity can be calculated by

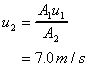
Notice how the downstream velocity only changes from the upstream by the ratio of the two areas of the pipe. As the area of the circular pipe is a function of the diameter we can reduce the calculation further,

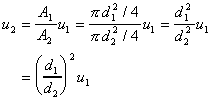
Now try this on a diffuser, a pipe which expands or diverges as in the figure below,

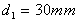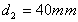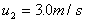If the diameter at section 1 is and at section 2 and the mean velocity at section 2 is . The velocity entering the diffuser is given by,

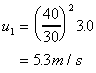
Another example of the use of the continuity principle is to determine the velocities in pipes coming from a junction.

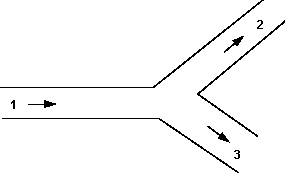
Total mass flow into the junction = Total mass flow out of the junction

r₁Q₁ = r₂Q₂ + r₃Q₃

When the flow is incompressible (e.g. if it is water) r₁ = r₂ = r

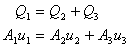
If pipe 1 diameter = 50mm, mean velocity 2m/s, pipe 2 diameter 40mm takes 30% of total discharge and pipe 3 diameter 60mm. What are the values of discharge and mean velocity in each pipe?